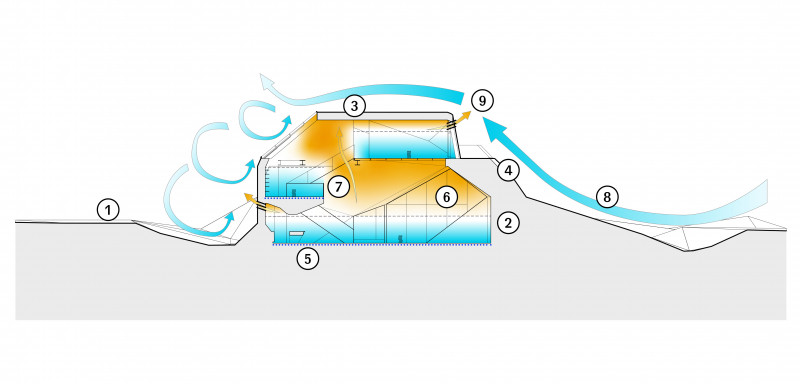
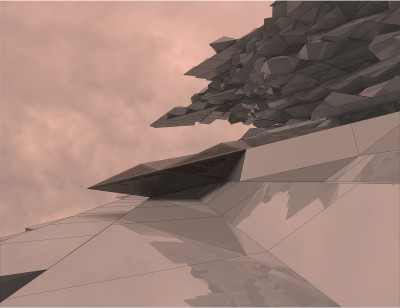
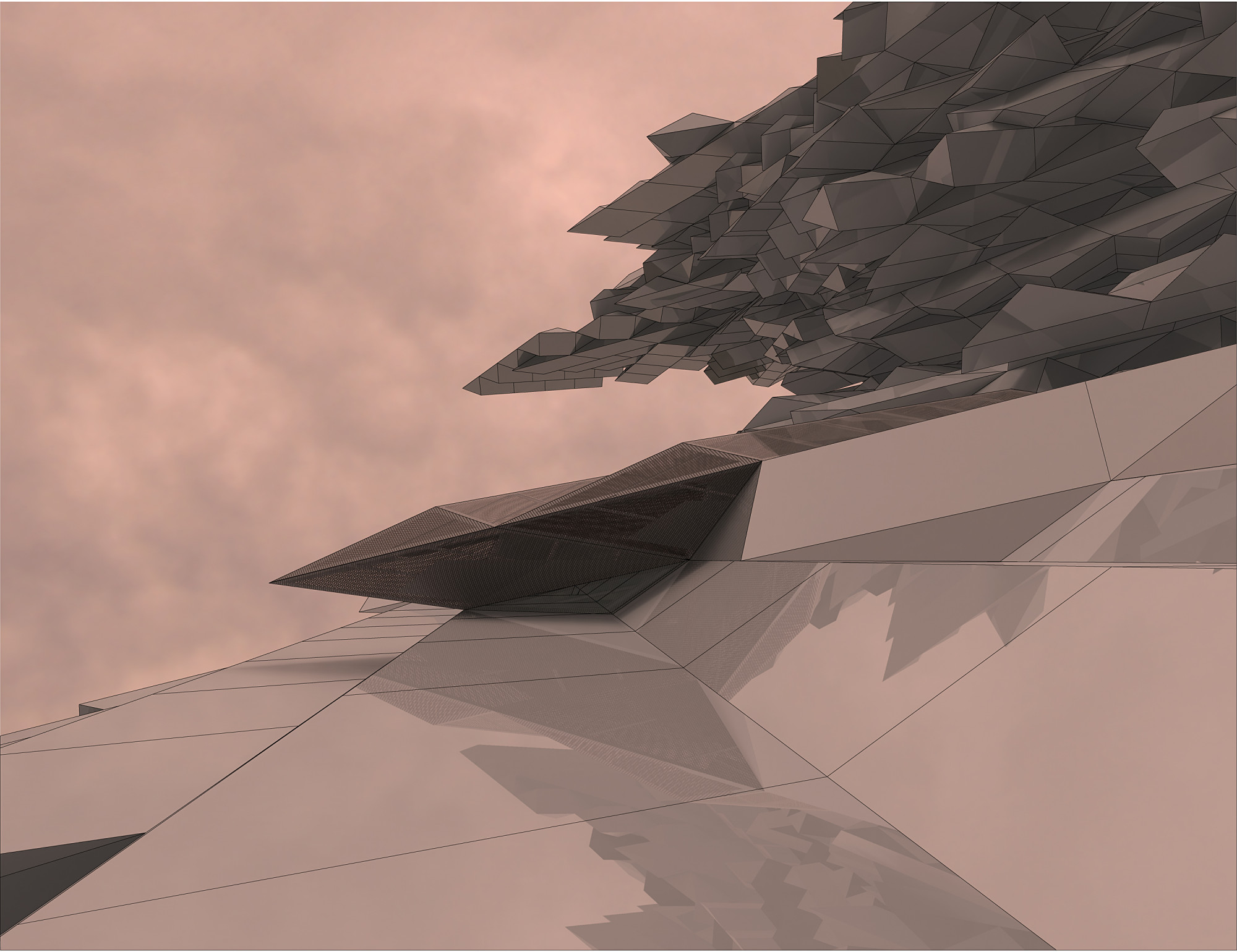
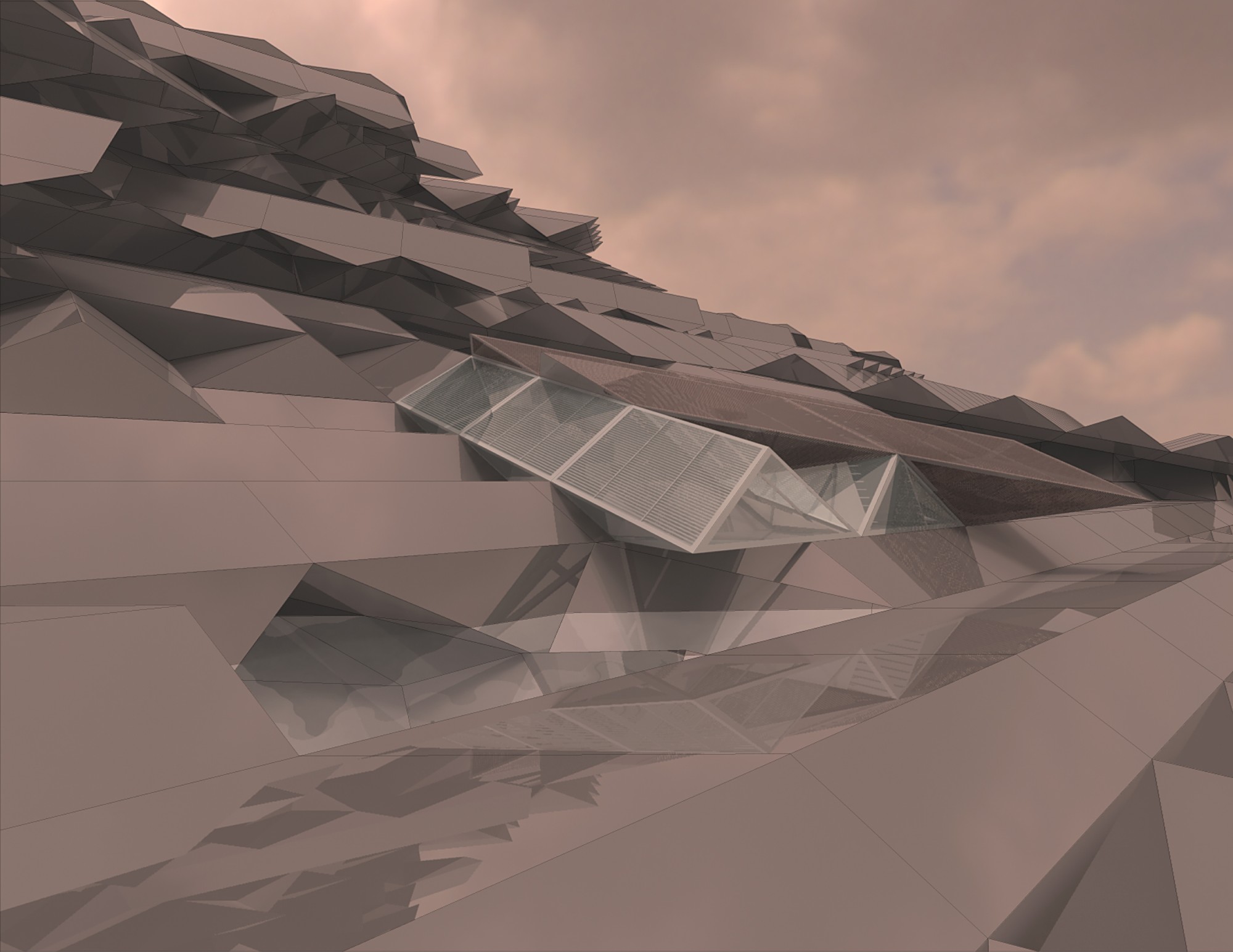
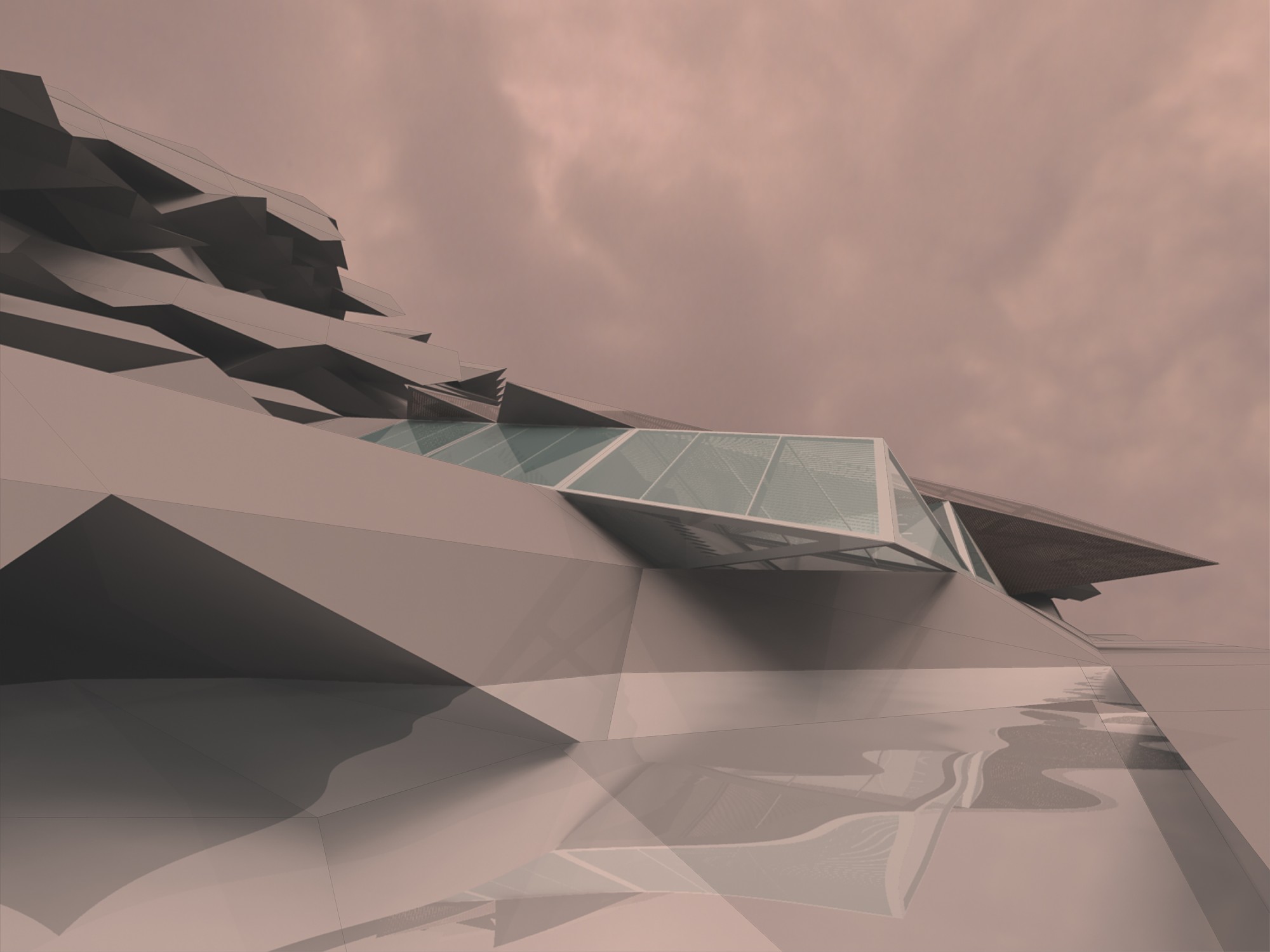
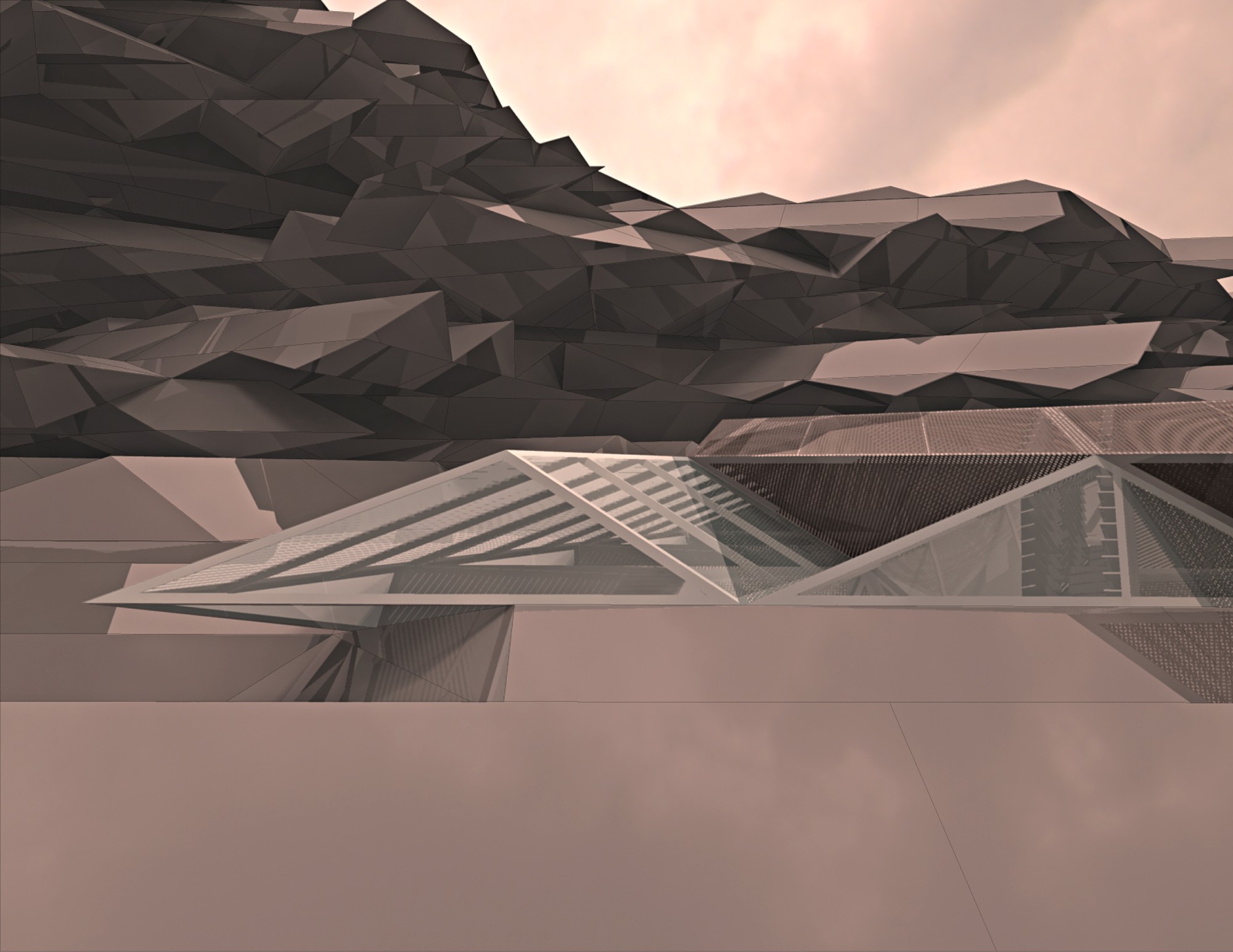
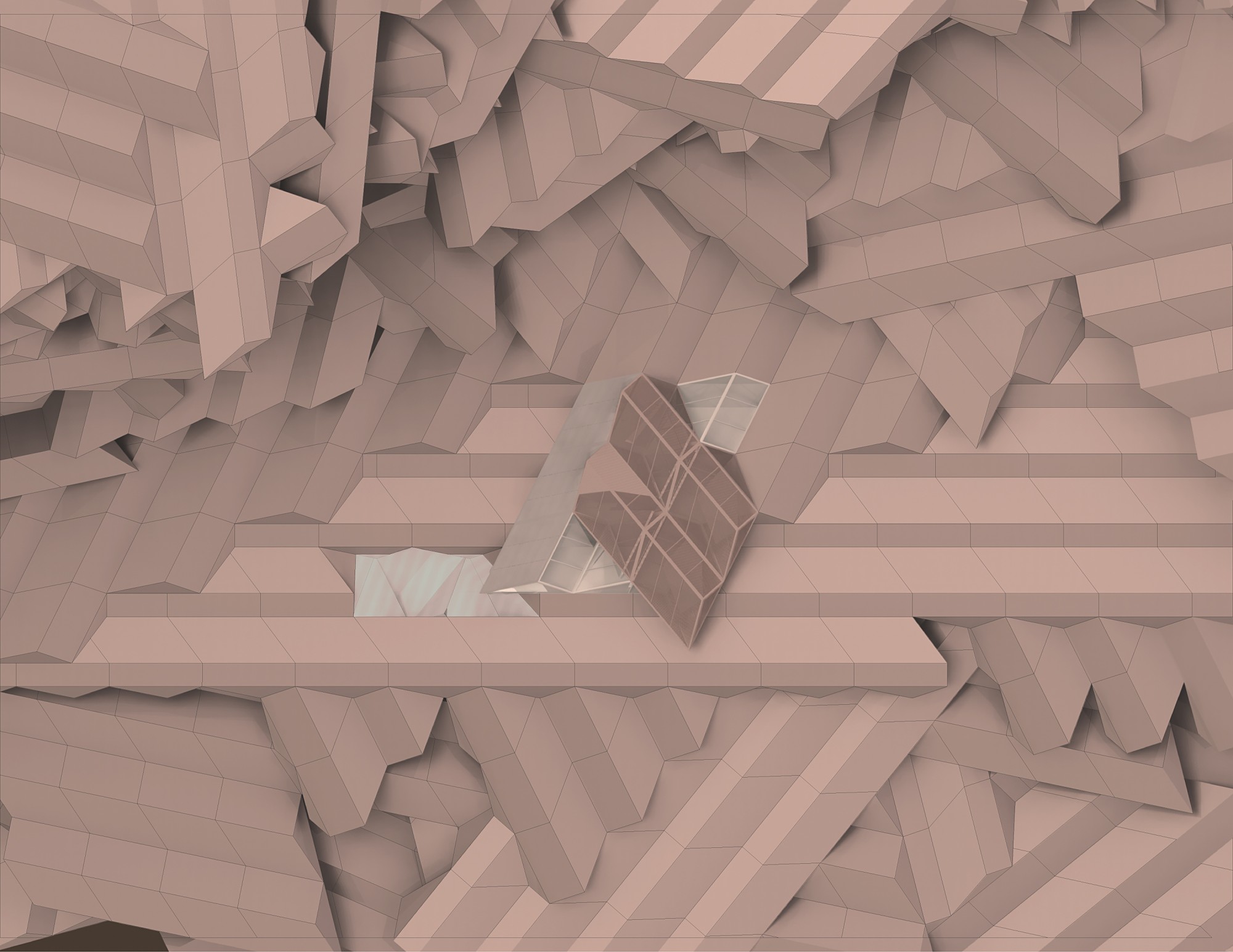
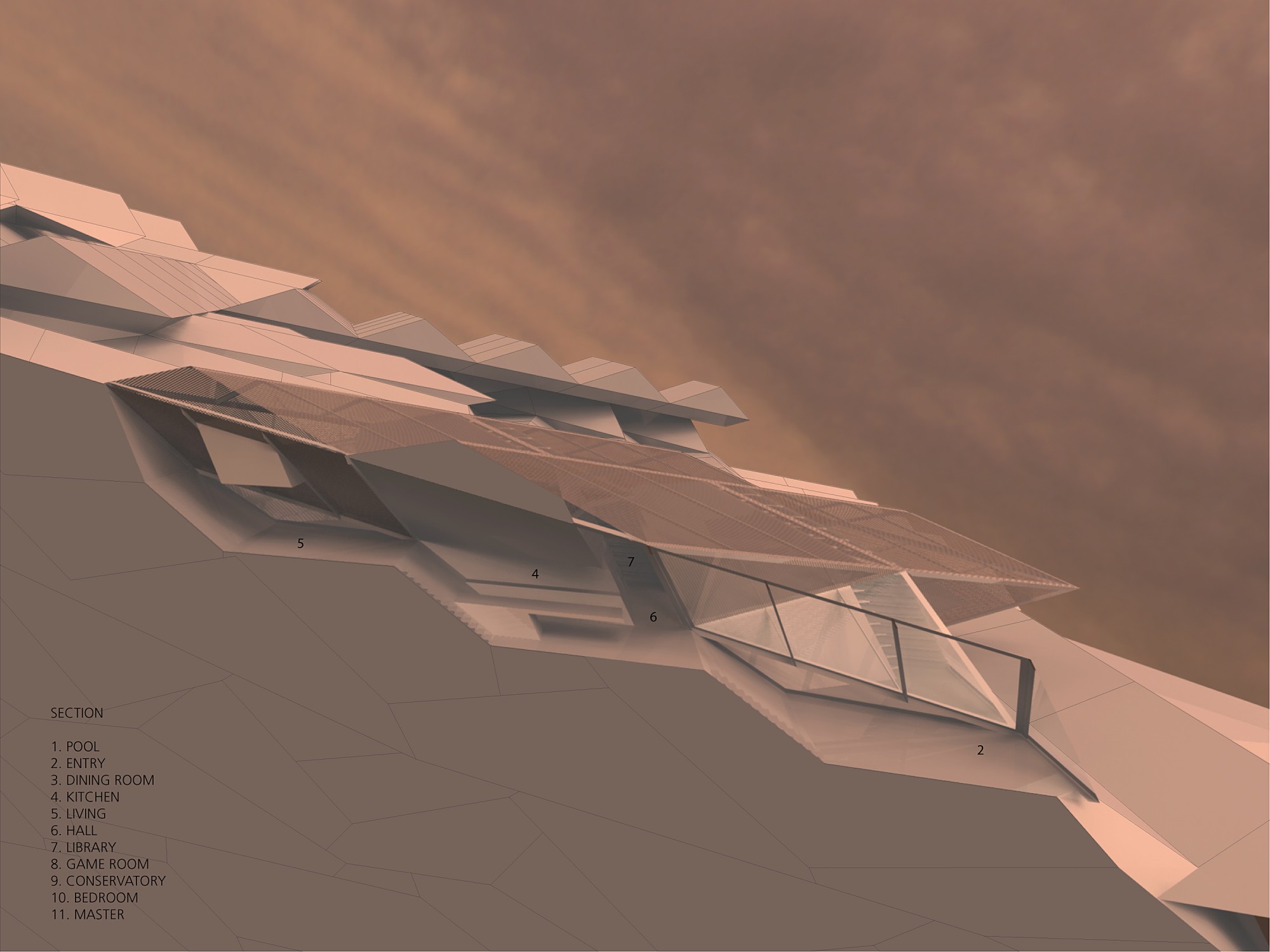
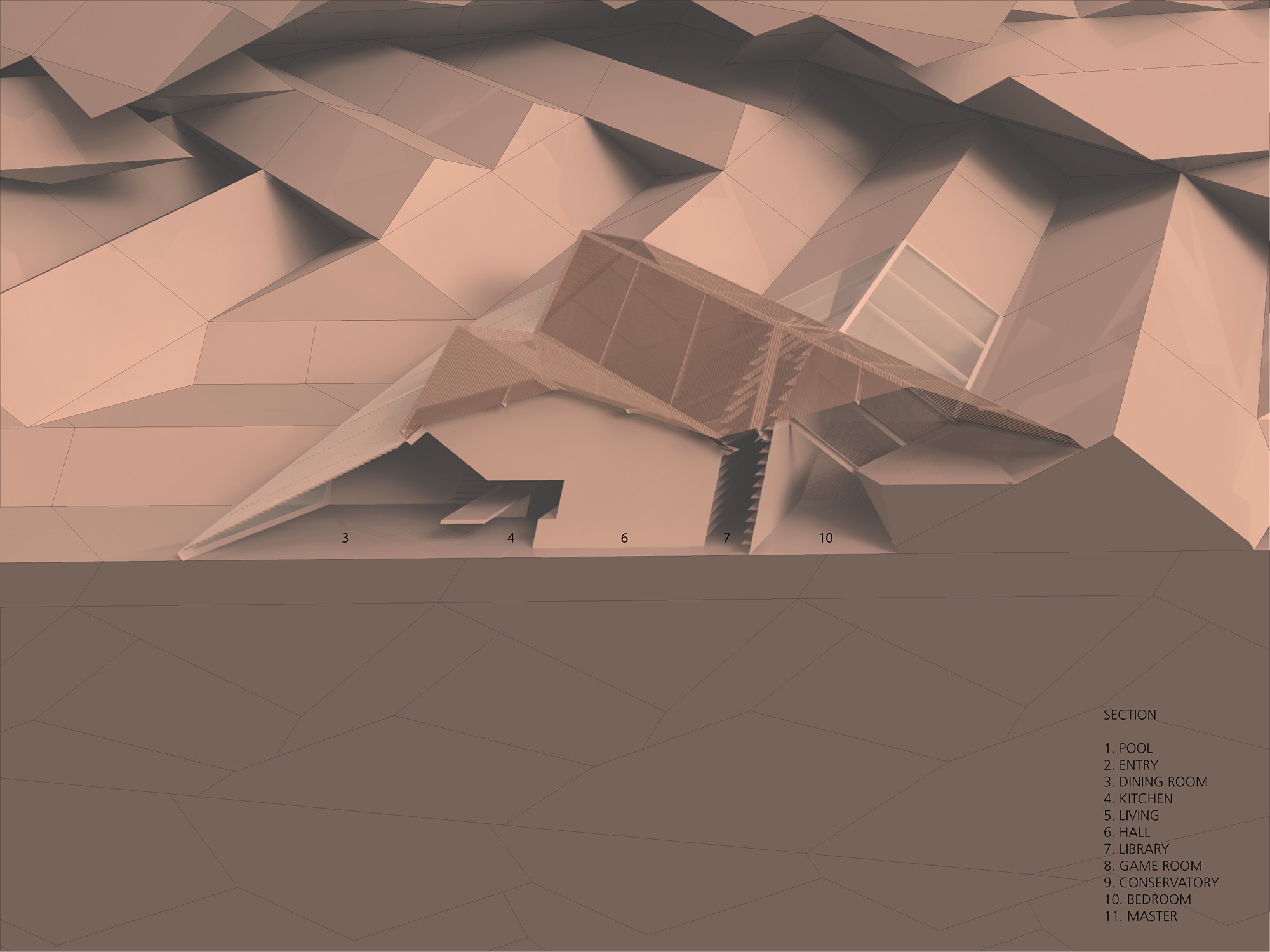
2024
California Houses
Thames & Hudson, London
2024
Nob Hill Gazette Vol. 46 No. 4
Clint Reilly Communications
2024
GA Houses 193
A.D.A. Edita Ltd., Tokyo
2023
GA Houses 191
A.D.A. Edita Ltd., Tokyo
2023
architizer.com
Material Bank
2023
architecturaldigest.com
Condé Nast, New York
2023
Acumen
Galerie Joseph, Paris
2023
wallpaper.com
T1 Media Ltd., London
2023
designboom.com
Design Boom S.r.l., Milan
2023
GA Houses 187
A.D.A. Edita Ltd., Tokyo
2022
Wallpaper 283
T1 Media Ltd., London
2022
domusweb.it
Editoriale Domus, Milan
2022
GA Houses 181
A.D.A. Edita Ltd., Tokyo
2022
azuremagazine.com
Azure Publishing, Toronto
2022
The New Minimalist Style
Loft, Barcelona
2021
dezeen.com
Dezeen Ltd., London
2021
Interior + Design 275
Forward Media Group, Moscow
2021
Frame 141
Frame Publishers, Amsterdam
2021
Traits D'Co
Pierrot Editions, Paris
2021
freeyork.org
Free York Inc., Wroclaw
2021
hypeandhyper.com
HH Ltd., Budapest
2021
designboom.com
Design Boom S.r.l., Milan
2021
contemporist.com
Mediavine Inc., Boca Raton
2021
ignant.com
Ignant GmbH, Berlin
2021
GA Houses 175
A.D.A. Edita Ltd., Tokyo
2021
AD Italia 473
Condè Nast, Milan
2021
Restless Living 63
Restless IVS, Copenhagen
2021
California Style
Monsa, Barcelona
2021
frameweb.com
Frame Publishers, Amsterdam
2021
admagazine.ru
Condè Nast, Moscow
2021
designboom.com
Design Boom S.r.l., Milan
2021
wallpaper.com
T1 Media Ltd., London
2020
GA Houses 173
A.D.A. Edita Ltd., Tokyo
2020
Interior + Design 267
Forward Media Group, Moscow
2020
designboom.com
Design Boom S.r.l., Milan
2020
GA Houses 169
A.D.A. Edita Ltd., Tokyo
2020
Villas Spring '20
Villas Decoration SPRL, Brussels
2020
Dwell March/April 2020
Dwell Life Inc., San Francisco
2020
GA Houses 167
A.D.A. Edita Ltd., Tokyo
2019
Secret Homes Season 1 Episode 3
Windfall Films Ltd., London
2019
Häuser 6|2019
Häuser, Berlin
2019
Secret Homes Season 1 Episode 2
Windfall Films Ltd., London
2019
Wallpaper* 239
T1 Media Ltd., London
2019
christiesrealestate.com
Christie's International, London
2019
Houses: Extraordinary Living
Phaidon, London
2019
Interni 691
Gruppo Mandadori, Milan
2019
Western Art & Architecture
JD Publishing, Bozeman
2019
The Contemporary House
Thames & Hudson, London
2019
GA Houses 163
A.D.A. Edita Ltd., Tokyo
2019
The Magazine 4
Minotti SpA, Milan
2019
Wallpaper* 238
T1 Media Ltd., London
2018
Homes for Our Time
Taschen, Cologne
2018
Il Nuovo Saggiatore
Italian Physics Society, Rome
2018
californiahomedesign.com
CDH Media, Los Angeles
2018
The New Urban House: A Global Survey
Yale University Press, New Haven
2018
AAG 2018
Klein Publishing GmbH, Berlin
2018
metropolismag.com
Sandow Media, Boca Raton
2018
wallpaper.com
T1 Media Ltd., London
2018
uncrate.com
Uncrate LLC, Columbus
2018
dezeen.com
Dezeen Ltd., London
2018
Dwell July/August 2018
Dwell Life Inc., San Francisco
2018
GA Houses 159
A.D.A. Edita Ltd., Tokyo
2018
Architecture Element 1: Entrance
Damdi, Seoul
2018
Architectural Element 2: Stairs
Damdi, Seoul
2018
Architectural Element 3: Roof
Damdi, Seoul
2017
The View 2|2017
Crew SF, San Francisco
2017
Mark 67
Frame Publishers, Amsterdam
2017
architectmagazine.com
Hanley Wood, Washington D.C.
2017
The University of Chicago Magazine 110
The University of Chicago, Chicago
2017
Private Gardens of the Bay Area
Monacelli, New York
2017
Maru 185
CA Press, Seoul
2017
Spaces 2.2
MIB Media, Chicago
2016
Container and Modular Buildings
DOM Publishers, Berlin
2015
30 Years of Emerging Voices
Princeton Architectural Press, New York
2015
The Fundamentals of Interior Architecture
Bloomsbury, London
2015
SFC&G 15|10
C&G Publications
2014
Who is the Architect?
Damdi, Seoul
2014
Mark 48
Frame Publishers, Amsterdam
2014
GA Houses 136
A.D.A. Edita Ltd., Tokyo
2014
Fast Company 185
Fast Company Inc., New York
2013
archleague.org
New York Architectural League, New York
2013
Platform 6
Actar, Barcelona
2013
New Container Architecture
Links International, Barcelona
2012
Digital Aptitudes
ACSA Press, Cambridge
2012
Mark 39
Frame Publishers, Amsterdam
2012
GA Houses 125
A.D.A. Edita Ltd., Tokyo
2012
Mobile Architecture: Construction and Design Manual
DOM Publishers, Berlin
2012
Architectural Record 12|02
BNP Media, Troy
2012
Roof Architecture + Design
Braun, Berlin
2012
Architectural Models
DOM Publishers, Berlin
2012
7x7 12|11
McEvoy Media, San Francisco
2011
Integration Through Computation
Acadia, Cambridge
2011
GA Houses 120
A.D.A. Edita Ltd., Tokyo
2011
San Francisco 11|12
Modern Luxury, San Francisco
2011
Remodeling
Damdi, Seoul
2011
Mobile Architecture
Damdi, Seoul
2011
Häuser 11|6
Häuser, Berlin
2010
Metropolis 10|4
Sandow Media, Boca Raton
2010
New York Times T Magazine 10|3
Arthur Ochs Sulzberger Jr., New York
2010
Dream Architecture
Edition Axel Menges, Berlin
2010
Architectural Models
Damdi, Seoul
2010
Sunset 10|3
Sunset Publishing, Menlo Park
2010
Ideat 78
Laurent Blanc, Paris
2009
California Home + Design 09|05
CHD Media, Los Angeles
2008
Wallpaper* 113
T1 Media Ltd., London
2008
Frameworks 08|Spring
UC Berkeley CED, Berkeley
2008
Young Architects Americas
Daab Pub, Berlin
2007
GA Houses 98
A.D.A. Edita Ltd., Tokyo
2007
Architecture of the San Francisco Bay Area: A History and Guide
William Stout Publishers, San Francisco
2007
Architectural Record 07|07
BNP Media, Troy
2007
The Magic of Small Spaces
Loft, Barcelona
2005
San Francisco 05|12
Modern Luxury, San Francisco
2002
GA Houses 70
A.D.A. Edita Ltd., Tokyo